Creeping Micropolar Flow past a Porous Oblate Spheroid
Abstract
A mathematical model for the creeping micropolar flow past a porous oblate spheroidal body is presented. The oblate spheroidal body possesses permeability k and uniform stream velocity is assumed far away from the body and along its axis of symmetry. The flow inside the particle obeys the Darcy- Brinkman laws of porosity and the fluid outside is governed by the A.C. Eringen micropolar flow theory. Drag is determined with varying values of the permeability parameter and the coupling number N. Also, the micropolar parameter m is studied numerically.
Keywords
Micropolar Fluid, Non-Newtonian, Porous, Oblate Spheroid
Introduction
Consider a spheroid S which is both oblate and porous. Let S be immersed in a fluid which is non-Newtonian in nature and where the outer fluid is governed by A.C Eringen's microfluid theory only [1]. The inner fluid, which is passing through spheroid S, takes into consideration the Darcy-Brinkman fluid model for porous mediums [2]. Fluid flow about S thus requires matching the general micropolar fluid model against the porous nature of S.
Microfluidic theory, in particular Micropolar fluid theory, is fascinating in its own right. It possesses an interesting ability to accurately portray fluids which enjoy their own microstructure; a characteristic completely lacked by classical fluid dynamical models. Real fluids with microstructure are especially important in industrial and engineering applications. In 1964 at the Naval Hydrodynamic conference in Bergen-Norway, Turkish-American engineering scientist, Professor Ahmed C. Eringen of Princeton University, presented his Microfluid theory [1]. The importance of his model is found in its generalisation of the classic Navier-Stokes equations while opening up the door to usage with complex fluids and creating higher applicability. As a sub-class of these fluids, he introduced the theory of Micropolar fluid [1] - which are physically represented as bar-like elements which possess micro-rotational effects and micro-rotational inertia. Applications include the solidification of liquid crystals, animals' blood, unusual lubricants and colloidal and suspension solutions.
Attention to the field of flows associated with porous mediums has been brought to the forefront with technological processes such as the flow in oil fields or reservoirs, filtration, spread of hazardous waste, petroleum exploration and recovery, bio-medical engineering and geology. Earlier studies of flow in porous media have revealed the Darcy laws, which relate linearly the flow velocity to the pressure gradient across a porous medium. Research into flow through porous media began with Hendrik C. Brinkman's 1947 paper 'A Calculation of Viscous Forces Exerted by a Flowing Fluid on a Dense Swarm of Particles' [2]. The underlying model was that of a spherical particle embedded within a porous mass. This flow was described by a modification of the Darcy equations, which was necessary to obtain consistent boundary conditions. A relation between permeability, particle size and density was obtained.
As a special case of an approximate sphere, the oblate spheroidal particle is studied, as it appears frequently in both nature and man-made objects. Examples of oblate spheroids include the planets Earth, Saturn and Jupiter, as well as flint pebbles and sea urchins. The confectionary Skittles™ and MnM's™, along with the popular children's toy, the Frisbee, are all popular oblate spheroids.
The years 1999-2002 gave noteworthy flow problems for through and past porous approximate spheres such as, 'Stokes Flow Inside a Porous Spherical Shell' by G.P. Raja Beckhar and T. Amaranath, as well as 'Axisymmetric Creeping Flow past a Porous Prolate Spheroid Particle using the Brinkman Model' by T. Zlatanovski [3] 2002 contributed 'Creeping Flow Past and Within a Permeable Spheroid' by P. Vainshtein, M. Shapiro and C. Gutfinger [4]. The current paper furthers the research done by Alexander, et al. in 2021 that provided exact solutions for the problem of micropolar flow past a porous approximate sphere [5]. The problem of 'Creeping Micropolar Flow past a Porous Oblate Spheroid is now presented.
Statement of the Problem
The solutions for the general case of a steady creeping micropolar fluid flow past a porous approximate sphere [5] are incorporated here. Due to the geometry of the problem spherical polar co-ordinates, are used. It is assumed that uniform velocity, U, exists far away from the approximate sphere and along the axis of symmetry , which coincides with the . It was also assumed that the fluid flowing outside of the porous approximate spheroid is Stokesian in nature, whereas the fluid flow within the porous region is governed by the Brinkman model for porous media flows [2].
The field equations for the region outside the porous approximate sphere are those governing the steady flow of an incompressible micropolar fluid under Stokesian assumption and in the absence of both body force and body couples. These external flow field equations are given as follows,
The internal flow field equations are those governing the motion of a fluid in a steady state within the porous medium and are based upon the Darcy-Brinkman model [2]. These field equations are,
where , and denote respectively the velocity vector, the microrotation vector and the fluid pressure with super-scripts signifying respective flows outside and inside of the porous approximate sphere. Furthermore, k represents the permeability of the porous medium and the material constants and satisfy the following inequalities:
Let the porous approximate sphere be centered at the origin and possess a radius of a. Such a body can be expressed as,
where,
[6] where is the Legendre function of the first kind and is called the Gegenbauer function of the first kind, each of order m. For simplicity considerations, regard the coefficient so adequately small that its squares and higher powers can be neglected.
The flow generated is axially symmetric about the and all flow functions are independent of . Hence, the velocity and microrotation vectors, are chosen in the form,
The Stokes stream function, , is introduced as follows:
as well as the following non-dimensional variables,
Equations (1)-(3) were non-dimensionalised, using (12), to give field equations for the region outside the approximate sphere as follows,
Equations (4)-(6) were non-dimensionalised, using (12), to give field equations for the region inside the approximate sphere as follows,
where, and are the axisymmetric Stokesian and Laplacian operators given respectively as,
and k refers to the permeability of the porous medium, N is the coupling number and m is the micropolar parameter given respectively by,
Eliminating pressure terms from equations (13)-(18) yielded the following expressions in terms of the stream functions,
where,
as well as expressions for the microrotation vectors, as,
The usual boundary conditions are considered: the continuity of the velocity components, pressure terms and tangential stress components, the hyperstick condition on the microrotation components, the imposition of regularity at infinity and velocity and pressure must remain non-singular at all points within the flow field, together with as and that is finite at
Consider the specific case of a porous oblate spheroidal body submerged within a creeping micropolar fluid, which is at rest far off at infinity.
The general equation of an of an approximate sphere is, [6]:
In other detailed analyses of the approximate sphere problem [5,6] it is shown that is sufficiently small so that orders of and higher can be neglected. Taking this into consideration and substituting into equation (25) yields an expression for an oblate spheroidal body of radius with the origin situated at the center [6]
Substitution of , into the principle equations governing the case of creeping micropolar flow past a porous approximate sphere will yield the various flow parameters of micropolar flow past in the case of a porous oblate spheroidal body.
Solution of the Outer Region
The following expressions represent the external fluid velocity vector, microrotation vector and pressure terms in the known case of Creeping Micropolar Flow past a Porous Approximate Sphere, [5]
In Equations (27)-(29), note that when is evaluated at . Substitutions of and are made into Equations (27)-(28). Let the subscript 'os' denote the case of a porous oblate spheroidal body. Note that contributes nothing due to the nature of the Gegenbauer function. The following equations, (30), (31) and (32), are the principle equations governing the external flow of a creeping Micropolar fluid past a porous oblate spheroidal body [7-10].
In each case,
where,
Solution of the Inner Region
The following expressions represent the internal fluid velocity vector, microrotation vector and pressure terms in the known case of Creeping Micropolar Flow past a Porous Approximate Sphere [5] are,
(34)
(35)
(36)
In Equation (34)-(36) above, note that when m ̄is evaluated at . Substitutions of and are made into Equations (34)-(36). Note that contributes nothing due to the nature of the Gegenbauer function. The following equations (37), (38) and (39) are the principle equations governing the internal flow of a creeping Micropolar fluid past a porous oblate spheroidal body,
In each case,
where,
Determination of drag
The compacted formula for Drag of a Creeping Micropolar Flow past a Porous Approximate Sphere [5] is,
When , , the resultant compacted drag formula is for micropolar flow past a porous oblate spheroidal body is,
where expressions for both and have been previously given.
Graphical work
In this section the dynamics of the drag of a creeping micropolar fluid past a porous oblate spheroid was observed in comparison to that of a creeping micropolar fluid past a porous perfect sphere and a non-porous perfect sphere in a Newtonian fluid.
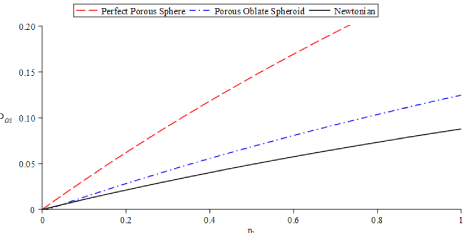
The drag formula (41) was plotted against its non-porous and Newtonian counterparts with permeability parameter for and for the value of and approximation parameter , as shown in Figure 1.
It can be observed that when approximation parameter, , and coupling numbers and , the drag of a creeping micropolar fluid past a porous oblate spheroidal body is lesser than that of its non-porous counterpart but greater than that of the classical Newtonian case. This is in accordance with proven micropolar and approximate sphere fluid theory.
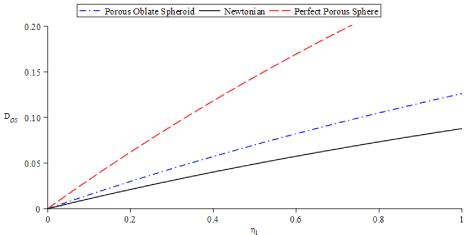
The drag formula (41) was plotted against its non-porous and Newtonian counterparts with permeability parameter for and for the value of and approximation parameter as shown in Figure 2.
It can be observed that when approximation parameter, , and coupling numbers and , the drag of a creeping micropolar fluid past a porous oblate spheroidal body is lesser than that of its non-porous counterpart but greater than that of the classical Newtonian case. This is in accordance with proven micropolar and approximate sphere fluid theory.
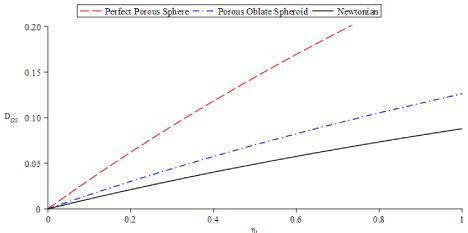
The drag formula (41) was plotted against its non-porous and Newtonian counterparts with permeability parameter for and for the value of and approximation parameter , as shown in Figure 3.
It can be observed that when approximation parameter, , and coupling numbers and , the drag of a creeping micropolar fluid past a porous oblate spheroidal body is lesser than that Of its non-porous counterpart but greater than that of the classical Newtonian case. This is in accordance with proven micropolar and approximate sphere fluid theory.
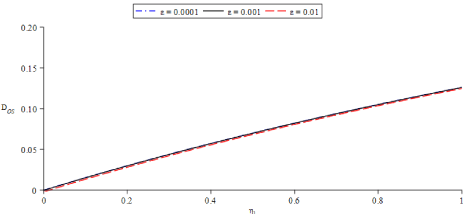
The final plot shows the drag formula for creeping flow of a micropolar fluid past a porous oblate spheroidal body (41) with permeability parameter for and for various values of approximation parameter . It can be observed from this plot that as the approximation parameter increases minutely, the drag holds steady. This is in accordance with known micropolar and approximate sphere fluid theory, as only and are incorporated in the drag of a porous oblate spheroidal body and hence the drag of the spheroid is fairly insensitive to the geometry of the problem. The implicit plot is given below in Figure 4.
Conclusion
Upon observation of the resultant drag formula, (41), for creeping micropolar flow past a porous oblate spheroidal body, the following observations were observed as central characteristic results.
I. It can be observed that as permeability increases, the drag on the porous oblate spheroidal body decreases.
II. As the coupling number N is decreasing, the drag on the porous oblate spheroidal body also decreases, when the fluid is micropolar.
III. The drag on the porous oblate spheroidal body, when the fluid is micropolar, is always greater than that of the known Newtonian case.
IV. As the coupling number m decreases, the drag on the porous oblate spheroidal body also decreases, when the fluid is micropolar.
V. Only the coefficients and contribute to the drag of a porous oblate spheroidal body in a creeping micropolar fluid. This implies that the drag on the porous oblate spheroidal body is relatively insensitive to the details of the surface geometry.
VI. As 𝜀 decreases minutely there is little to no change in the drag of the porous oblate spheroidal particle in a creeping micropolar fluid. Again, this implies that the drag on the oblate spheroidal body, when the fluid is micropolar, is relatively insensitive to the details of the surface geometry.
VII. The drag on the porous sphere is more than that of the drag on the porous oblate spheroidal body in the presence of a creeping micropolar fluid.
References
- Eringen AC (1966) Theory of Micropolar Fluids. J Math Mech 16: 1-18.
- Brinkman HC (1947) A Calculation of Viscous Force Exerted by a Folowing Field on Dense Swarm of Particles. Applied Scientific Research A1: 27-34.
- Zlatanovski T (1999) Axisymmetric Creeping Flow Past a Porous Prolate Spheroidal Particle using the Brinkman Model. J Mech Appl Math 52: 111-126.
- Vainshtein P, Shapiro M, Gutfinger C (2002) Creeping Flow Past and Within a Permeable Spheroid. International Journal of Multiphase Flow 28: 1945-1963.
- Alexander R, Rahaman K, Commissiong DMG (2021) Creeping Micropolar Flow past a Porous Approximate Sphere. International Research Journal of Modernization in Engineering Technology & Science 3: 15-26.
- Ramkissoon H (1997) Polar Flow Past a Spheroid. Engineering Transactions 45: 171-180.
- Lukaszewicz, Grzegorz (1999) Micropolar Fluids: Theory and Applications.
- Majmudar SR, Ramkissoon H (1977) Axisymmetric Creeping Flow of a Micropolar Fluid. The Tensor 31: 107-117.
- Ramkissoon H, Majumdar SR (1975) Creeping Flow of a Micropolar Fluid Past a Sphere. Letters in Applied and Engineering Sciences 3: 133-142.
- Srinivasacharya D, Rajyalakshmi I (2004) Creeping Flow of Micropolar Fluid Past a Porous Sphere. Applied Mathematics and Computation 153: 843-854.
Corresponding Author
K Rahaman, The University of the West Indies, St. Augustine Campus, WI, Trinidad and Tobago.
Copyright
© 2022 Alexander R. This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited.